There are several known examples of dcpos that are well-filtered, but not sober, and I have mentioned a few ones here, where I have made a detailed description of one due to Xiaodong Jia. I would like to explain another one, due to Dongsheng Zhao, Xiaoyong Xi, and Yixiang Chen [1]. This is a very simple modification of Johnstone’s non-sober dcpo J [2]. Contrarily to Xiaodong Jia’s dcpo (and to J), it is uncountable, but it may be easier to see why it must be well-filtered.
Let me remind you that a space is well-filtered if and only if for every open subset U, for every filtered family (Qi)i ∈ I of compact saturated subsets whose intersection is included in U, some Qi is already included in U. Every sober space is well-filtered, but the converse fails. (See Section 8.3.1 of the book for further information.)
The cofinite and the cocountable topologies
Before we recall Johnstone’s dcpo J and its modification by Zhao, Xi, and Chen, we should first examine the difference between two funny topologies, the cofinite topology, and the cocountable topology.
The reason why I am starting with this is that J has a so-called top row, and that the induced topology on that top row is the cofinite topology. The dcpo constructed by Zhao, Xi, and Chen has a similar shape, but aims to obtain the related, but in fact rather different, cocountable topology on the top row. Considering that I have seen Dongsheng Zhao use the cocountable topology as a counterexample in several papers, I would imagine that this is the way they came up with the construction of [1].
The cofinite topology on a set X is defined by letting the closed sets be exactly the finite subsets of X, plus X itself. If X is finite, that is just the discrete topology, and that is not specially interesting. In general, this builds a topological space that is T1 but not T2. It is Noetherian, meaning that all its subsets are compact (Exercise 4.4.24 of the book); when X is infinite, it is not sober (Example 8.2.8 in the book—although only the case of N is dealt with there, the argument is similar for any infinite set X); and, when X is infinite again, it is not well-filtered. Why? Because the family of all non-empty open subsets of X is a filtered family of compact saturated subsets (X is Noetherian), whose intersection is included in the open set ∅, but no element of the family is included in ∅.
The cocountable topology is defined similarly: its closed sets are exactly the (finite or infinite) countable subsets, plus the whole set X itself. If X is countable, this is just the discrete topology, so only the case where X is uncountable is really interesting. Whatever the case is, any set X with the cocountable topology is T1 but not T2.
However, there is a big difference with the cofinite topology: in the cofinite topology, we had an incredible amount of compact subsets (all sets were compact); in the cocountable topology, there are very few of them:
Lemma. On a set X with the cocountable topology, the compact subsets are exactly the finite subsets.
Proof. Every finite subset is always compact. Conversely, let K be a compact subset of X, and let us assume that K is infinite. Then there is an infinite sequence x0, x1, …, xn, … of pairwise distinct points in K. Let Cn be the set {xn, xn+1, …}. This is a countable set, hence a closed set. Each Cn intersects K, and the sets Cn form a filtered family (in fact a decreasing sequence) of closed sets. Since K is compact, the intersection ∩n ∈ N Cn must also intersect K… but ∩n ∈ N Cn is empty: contradiction. ☐
It follows that any set with the cocountable topology is well-filtered: a filtered family (Qi)i ∈ I of compact saturated sets is just a filtered family of finite subsets, and its intersection Q must therefore be one of the sets Qi already. Hence, if Q is included in some open subset U, then Qi is trivially also included in U. We obtain the following.
Proposition A. Given any set X, the space X with the cocountable topology is well-filtered. If X is uncountable, then it is not sober.
Proof. We have just proved well-filteredness. As far as sobriety is concerned, the union of any two proper closed subsets (hence, countable subsets) is countable, hence proper (because X is uncountable; “proper” means different from the whole set X). Passing to complements, any two non-empty open subsets have a non-empty intersection. We claim that this says that X is itself an irreducible closed set: for any two open subsets U and V that intersect X (i.e., that are non-empty), U ∩ V also intersects X (is non-empty). Clearly, X has no largest element (it is T1, so its specialization ordering is equality), or equivalently is not the closure of a point, so X is not sober. ☐
Johnstone’s dcpo J
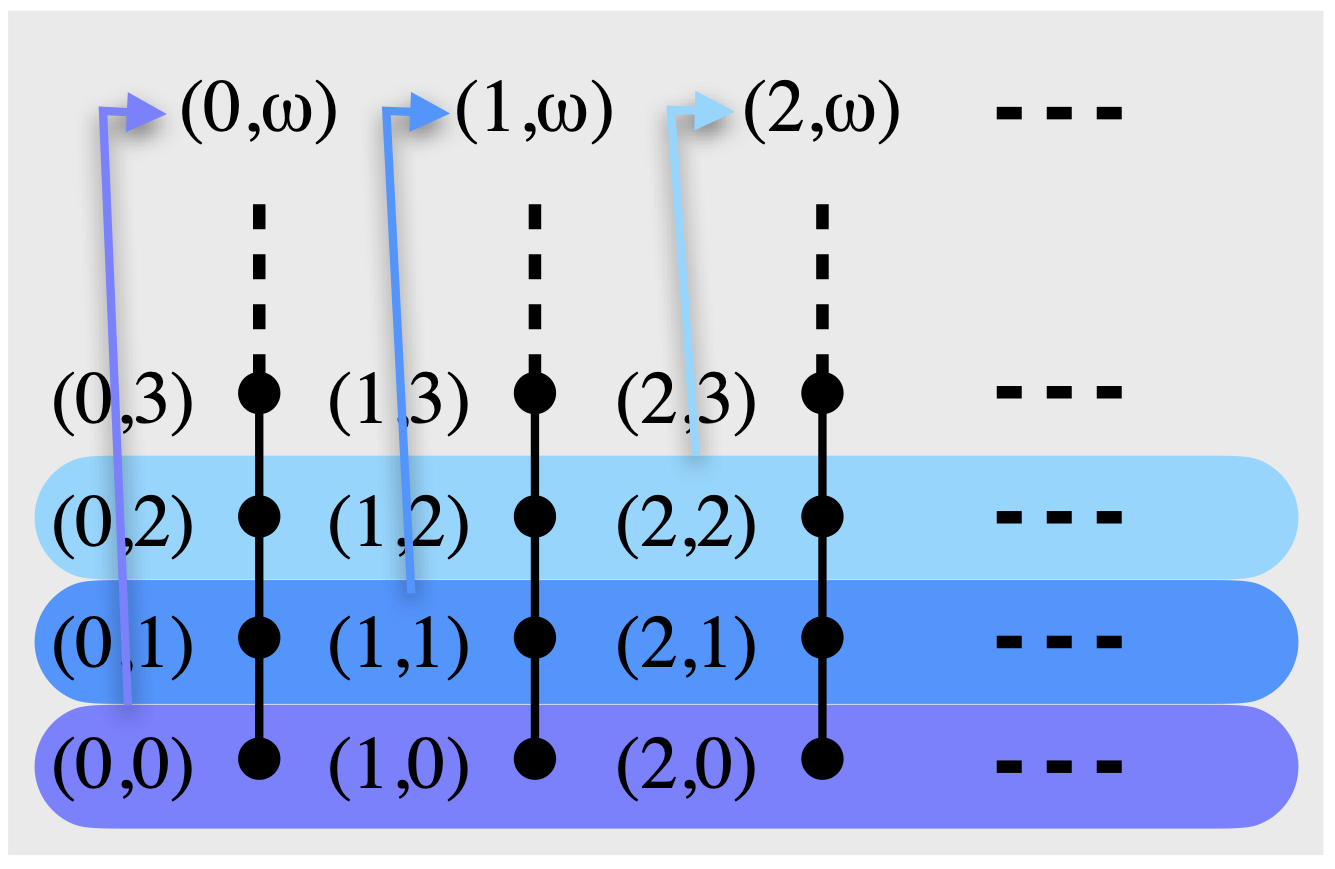
Let me recall how J is defined. J is the set of pairs (j, k) in N × (N ∪ {ω}), ordered by (j, k) ≤ (j’, k’) if and only if either j=j’ and k≤k’, or k≤j’ and k’=ω. In pictures, this is the set of points displayed below. To go up in the Johnstone ordering, you can either go up vertically, following one of the black lines; or you can follow one of the colored arrows right to the top (for example, if you are on the third row from the bottom, colored with the lightest shade of blue, you can move right to the point (2,ω ).)
The Zhao-Xi-Chen dcpo Jα is defined similarly, except that N is replaced by a regular ordinal α. (I will explain what this is in a jiffy.) J is obtained when α=ω, the first infinite ordinal. The interesting new case, which is the one considered by Zhao, Xi, and Chen, is when α is the first uncountable ordinal ω1, where something different happens, just like as in the cofinite/cocountable situation.
Ordinals, regular ordinals
In the sequel, let me remind you that α, as an ordinal, is itself a poset of ordinals, namely the ordered set of ordinals strictly less than α. For example, 0 is the empty set, 1 = {0}, 2 = {0, 1}, etc. Those are the finite ordinals, but we can go on way further: the first infinite ordinal is ω={0, 1, 2, …}, then we find ω+1={0, 1, 2, …, ω}, ω+2={0, 1, 2, …, ω, ω+1}, and so on. And those are only very low, countable ordinals.
With this encoding, for any two ordinals α and β, we say that α is strictly smaller than β (α<β) if and only if α is an element of β, seen as a set; α is smaller than or equal to β (α≤β) if and only if α<β or α=β, and that is equivalent to α⊆β. (Refer to the above examples, in order to check this on finite ordinals 0, 1, 2, …, if you don’t already know how this works.)
The limit of any chain of ordinals is their supremum, and since ≤ is just inclusion, it should come as no surprise that the limit is just the union.
With those definitions, one might guess that the ordinals that are dcpos are exactly the limit ordinals… but that is just the other way around! For example, the limit ordinal ω={0, 1, 2, …} is not a dcpo, because its supremum ω fails to be in it.
The ordinals that are dcpos are exactly those that are not limit ordinals. For example, 0, 1, 2, …, ω+1, ω+2, …, are all dcpos. The interesting ones are those of the form α+1, where α is a limit ordinal: α+1 is the dcpo of all ordinals less than or equal to α, and in particular α is its largest element. We will need to keep that in mind when we see the description of the Zhao-Xi-Chen dcpo Jα below.
We will be specifically interested in certain ordinals called regular ordinals. A regular ordinal is a limit ordinal α such that, for every ordinal γ<α, for every monotonic chain (βi)i<γ of ordinals βi<α, supi<γ βi is again strictly less than α. In other words, the supremum of any small family of small ordinals is again small, where by small I mean strictly less than α, or (for the whole family) indexed by a small ordinal.
The first regular ordinal is ω. In that case, small means finite, and indeed the supremum of any finite collection of finite ordinals (natural numbers) is a finite ordinal.
The one that Zhao, Xi, and Chen consider is ω1, namely the first uncountable ordinal. In that case, small means countable, and indeed the supremum of any countable collection of countable ordinals is countable. In other words, the union of countably many countable ordinals (or even sets) is countable.
There are other regular ordinals, but knowing that ω and ω1 are regular ordinals will be enough for our purposes.
The dcpo Jα
Let me give the actual definition of Jα, where α is any limit ordinal. (We do not require it to be a regular ordinal yet.)
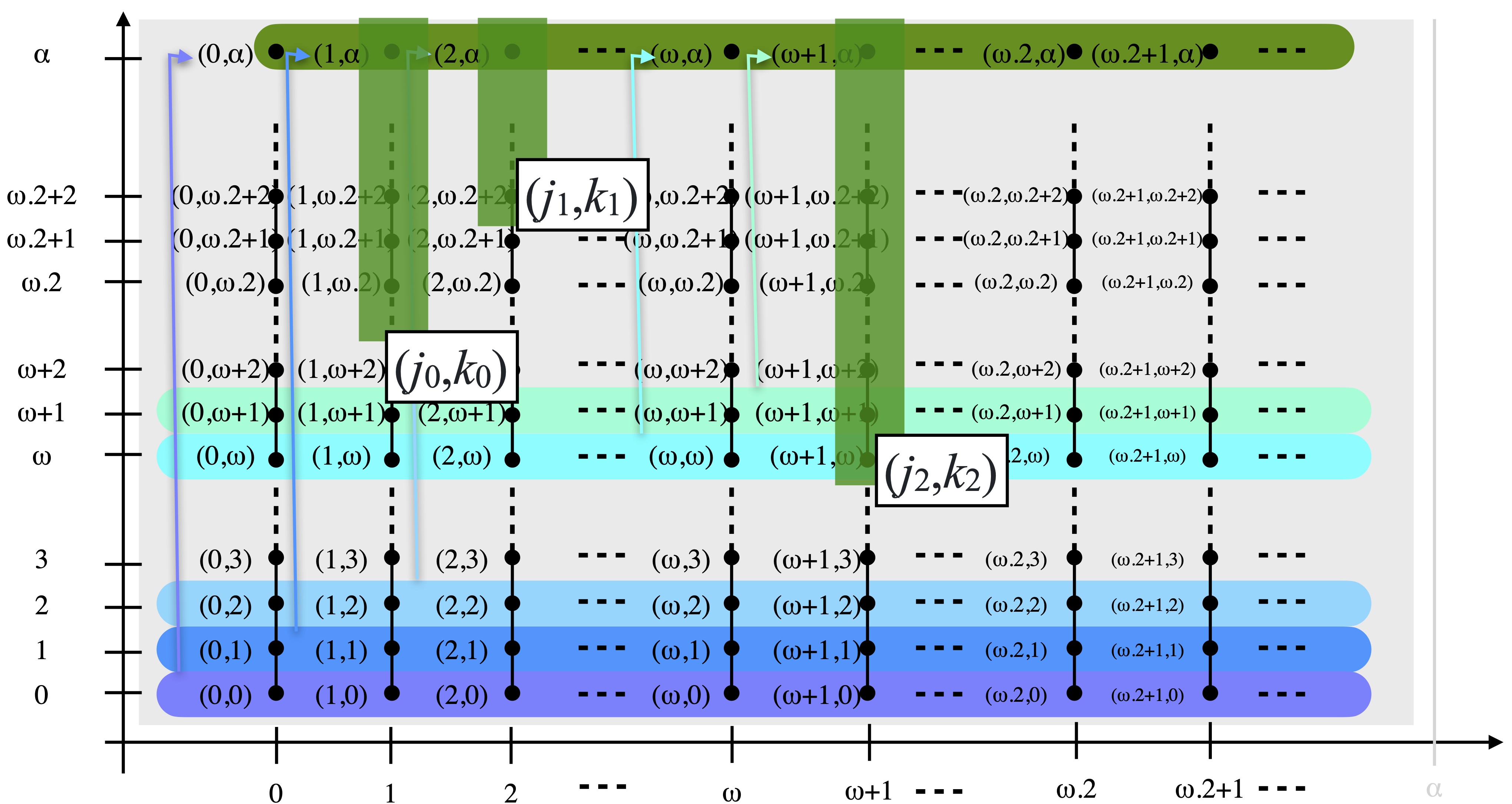
Jα is the set of pairs (j, k) in α × (α+1), ordered by (j, k) ≤ (j’, k’) if and only if either j=j’ and k≤k’, or k≤j’ and k’=α. See the picture below, and compare with the picture given for J above. To go up in Jα, you can either go up vertically, following one of the black lines; or you can follow one of the colored arrows right to the top.
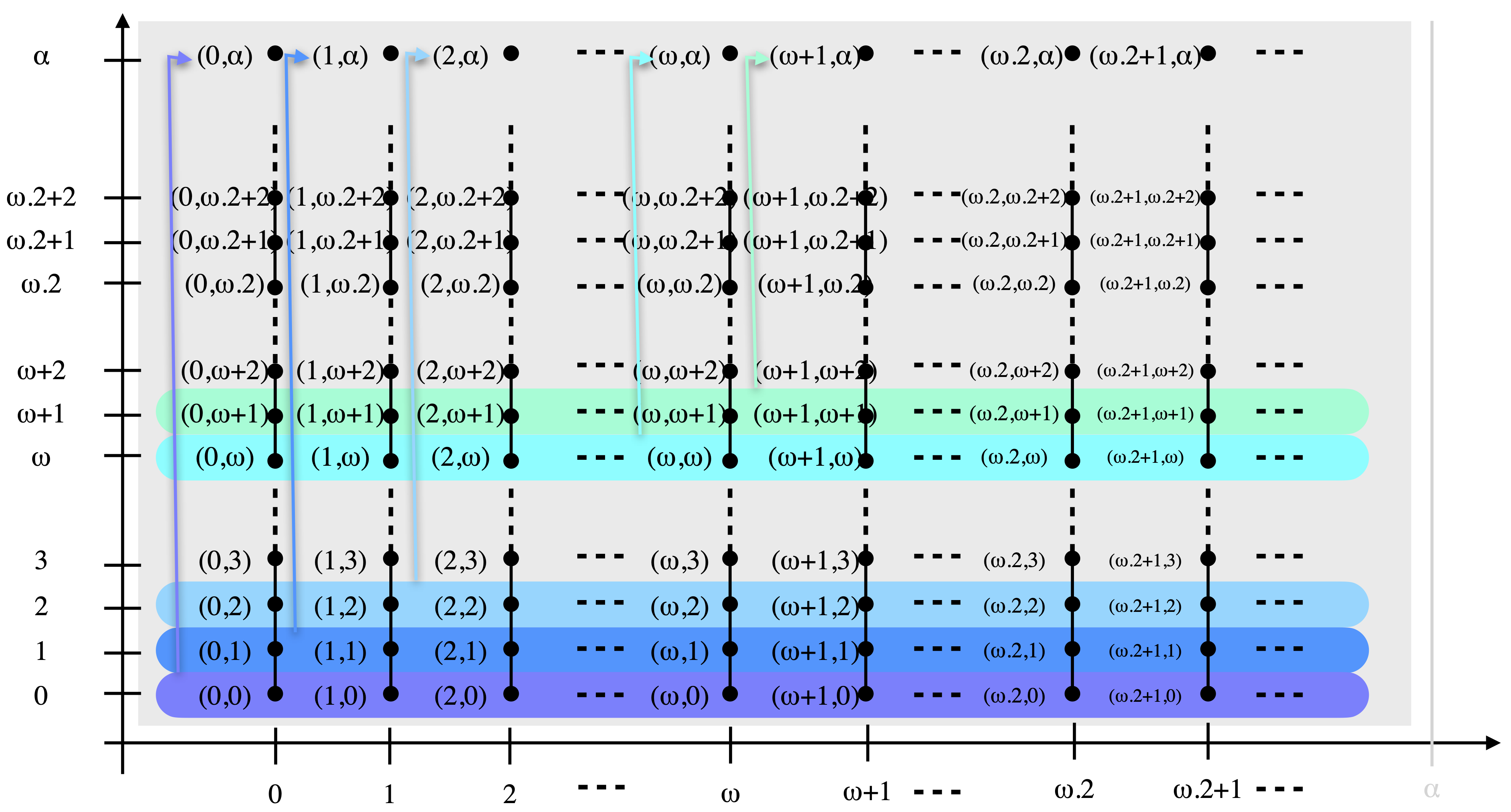
This is very similar to J. In fact, J is just Jω, of course. When α>ω, beware that there are a few differences. The most notable one, for now, is that, in J, all the chains (non-empty totally ordered families) are either trivial (contain their supremum), or their supremum is on the top row. In Jα where α>ω, however, the chain of elements (0,0), (0,1), (0,2), …, has (0,ω) as supremum, and that is not on the top row.
At least, the shape of non-trivial directed families is simple. Just as in J, those are just chains included in one of the columns. (Zhao, Xi, and Chen call pillars those columns.) We require α to be a limit ordinal, so that every element of the top row is a limit of a chain of elements strictly below it.
This allows us to use the following familiar argument to discover some of the properties of open subsets of Jα, just as in J. Let U be an open subset of Jα, and let us imagine that U contains a point (j,α) on the top row of Jα. Since (j,α) is the supremum of the chain of elements (j,k) with k<α, at least one of these elements (j,k) is in U. Since U is upwards-closed, all the points (j’,α) with j’≥k are in U. In other words, all of the elements of the top row to the right of (k,α) are in U.
As a first, easy consequence, if we consider any two non-empty open subsets U and V of Jα, then their intersection must be non-empty. Indeed, we pick a point of U on the top row of Jα (we are in a dcpo, and in a dcpo every element lies below some maximal element, by Zorn’s Lemma), and then all the points (j’,α) with j’≥k are in U, for some k<α. Similarly, all the points (j’,α) with j’≥k‘ are in V, for some k’<α. Then max(k,k’)<α (because max(k,k’) is equal to k or to k’), and all the points (j’,α) with j’≥max(k,k’) are in both U and V.
It follows that Jα is itself an irreducible closed set, in the sense that for any two open subsets U and V that intersect Jα (i.e., that are non-empty), U ∩ V also intersects Jα (is non-empty). Clearly, Jα has no largest element, or equivalently is not the closure of a point, so:
Fact 0. For every limit ordinal α, Jα is a non-sober dcpo.
The induced topology on the top row
The induced topology on the top row Max J of J is the cofinite topology, as I have already mentioned. (This is Exercise 8.2.14 in the book.) What is the induced topology on the top row Max Jα of Jα, for an arbitrary regular ordinal α?
We first consider any non-empty open subset U of Max Jα. Then U = V ∩ Max Jα for some non-empty Scott-open subset V of Jα. Since U is non-empty, it contains a point (j,α). As before, since (j,α) is the supremum of the chain of elements (j,k), k<α, at least one of these elements (j,k) is in V. Since V is upwards-closed, all the points (j’,α) with j’≥k are in V, and also in Max Jα, therefore in U.
This implies that Max Jα – U is small, in the sense that we have used earlier: we can enumerate its elements by using only the elements of some ordinal k strictly less than α. It will be practical to make that notion more precise, and more suited to the special case of Max Jα, as follows.
Let me say that a subset C of Max Jα is Jα–small if and only if there is an ordinal k<α such that all the points (j’,α) of C are such that j’<k. (Small and Jα–small are really equivalent notions, but I do not want to have to justify this.) We have just seen that every proper closed subset of Jα is Jα–small.
For example, when α=ω, this means that Max Jα – U is finite (whereas Max Jα is infinite), hence that U is open in the cofinite topology, as we expected. When α=ω1, this means that Max Jα – U is countable (whereas Max Jα is uncountable), namely that U is open in the cocountable topology.
In general, let us call cosmall topology the topology on Max Jα whose closed subsets are the whole set, plus all the Jα-small subsets C. This is really a topology. It is easy to see that any intersection of closed sets is closed, and finite unions of closed sets are closed: in fact, since α is a regular ordinal, any union of a small family of closed sets (where small means indexed by some ordinal strictly less than α, as before) is again closed.
The following should now be expected.
Lemma B. For every regular ordinal α, the induced topology on Max Jα is the cosmall topology. In other words, its proper closed subsets are exactly the Jα-small subsets.
Proof. We have seen that every closed set is Jα-small. It remains to show that, conversely, every Jα-small subset C of Max Jα is closed.
Since C is Jα-small, by definition, there an ordinal k0<α such that all the points (j’,α) of C are such that j’<k0. Note that k0+1<α: otherwise we would have k0+1=α, which is impossible since α is a limit ordinal, and k0+1 is not.
In order to show that C is closed in Max Jα, we must exhibit a Scott-closed subset C’ of Jα such that C’ ∩ Max Jα = C. We start by taking the downward closure ↓C of C in Jα, but that may fail to be closed under directed suprema. Instead of closing it under directed suprema, and following Zhao, Xi, and Chen, we do a much simpler thing: we add all the points (j,k) in Jα such that k≤k0. In other words, we define C’ as ↓C ∪ (α × (k0+1)).
Note that, since k0+1<α, no element of α × (k0+1) is on the top row; hence the elements of C’ that are on the top row Max Jα are exactly those of C. In pictures, C’ looks like the following. (C is in red, on top, α × (k0+1) is shown in light pink, and the dark pink area is the part of ↓C that is not covered by the other two areas, namely by α × (k0+1) or by C itself.)
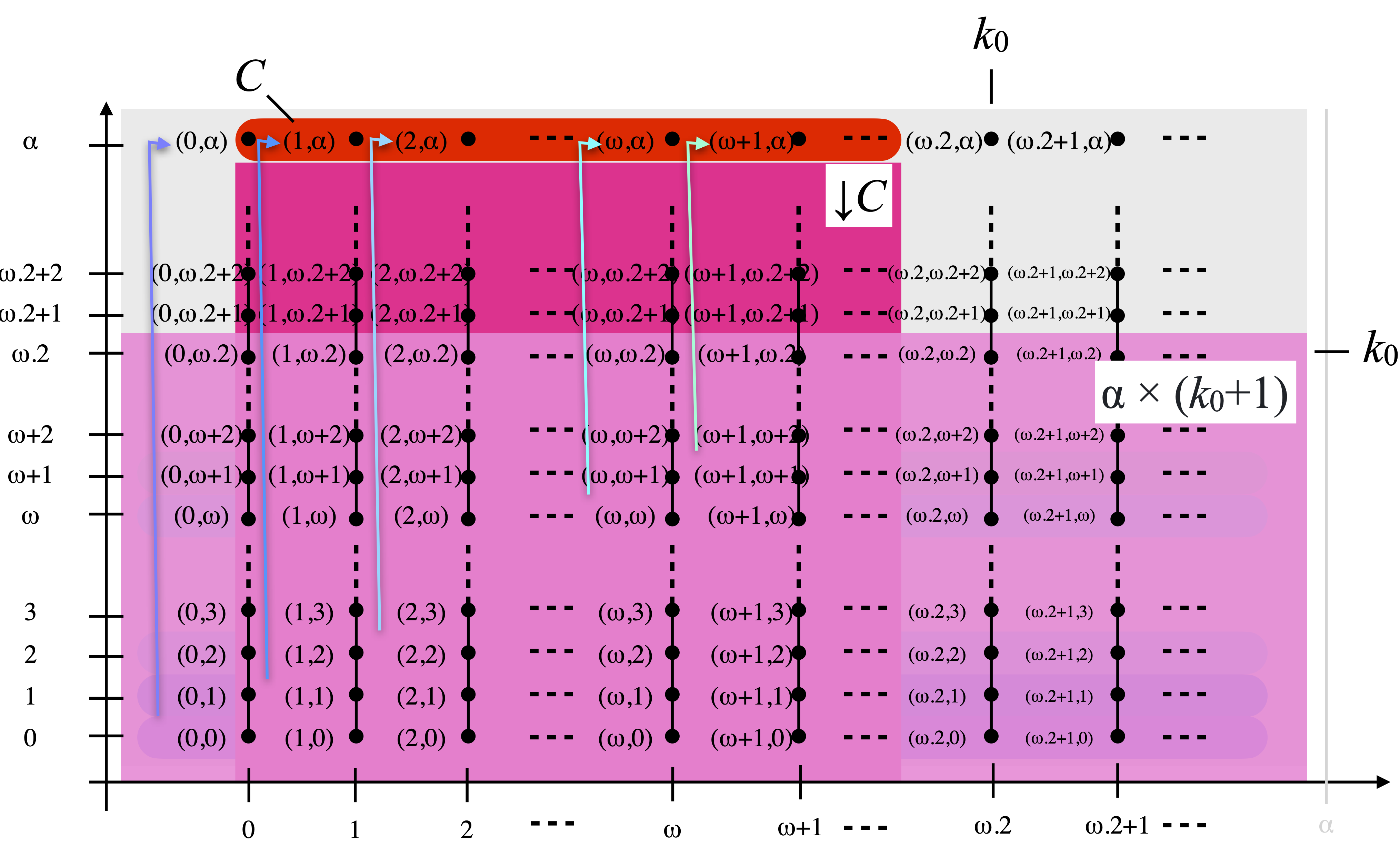
The reason why we add α × (k0+1) to ↓C in the definition of C’ is that the shape of ↓C is more complicated than what the picture above may suggest. For every point (j’,α) of C (with j’<k0), we will find the whole of ↓(j’,α) inside ↓C, and that not only includes column number j’ (right below (j’,α), vertically) but also the whole row number j’, and consequently all the rows below it as well. Hence ↓C really contains not only the red and dark pink areas above, but most of the light pink area as well. The light pink area α × (k0+1) is slightly larger, and is designed to make sure that C’ is Scott-closed.
Let us show this. First, C’ is downwards-closed. This is obvious from the definition of C’, which is the union of two downwards-closed sets. Next, we consider any non-trivial directed family D of elements of C’. That is completely included in some column, say number j. If D contains an element (j,k) where k≥k0+1, then D must extend into the dark pink or red areas, and then sup D is again in ↓C, hence in C’. Otherwise, D is entirely included in α × (k0+1), which is already a dcpo (under the ordering (= × ≤)), because k0+1 is not a limit ordinal: recall that the ordinals that are dcpos are exactly those that are not limit ordinals!
To sum up, C’ is Scott-closed, its intersection with Max Jα is C, hence we are done. ☐
This is interesting, because of the following analogue of Proposition A.
Proposition A’. For every regular ordinal α>ω, Max Jα (with the induced, namely with the cosmall topology) is well-filtered.
Proof. The proof is as for Proposition A: we simply show that all the compact subsets are finite. Imagine that we had an infinite compact subset K of Max Jα. We pick an infinite sequence (j0,α), (j1,α), …, (jn,α), … of pairwise distinct points in it, we define the set Cn as {(jn,α), (jn+1,α), …}. This is a countable set, hence a set of points indexed by indices in ω, and ω<α by assumption. Hence Cn is Jα-small, and therefore closed. We have obtained a decreasing sequence of closed sets that each intersect K, but whose intersection (being empty) does not intersect K: contradiction. ☐
The compact saturated subsets of Jα
In order to examine whether Jα is well-filtered or not, we need to examine its compact saturated subsets.
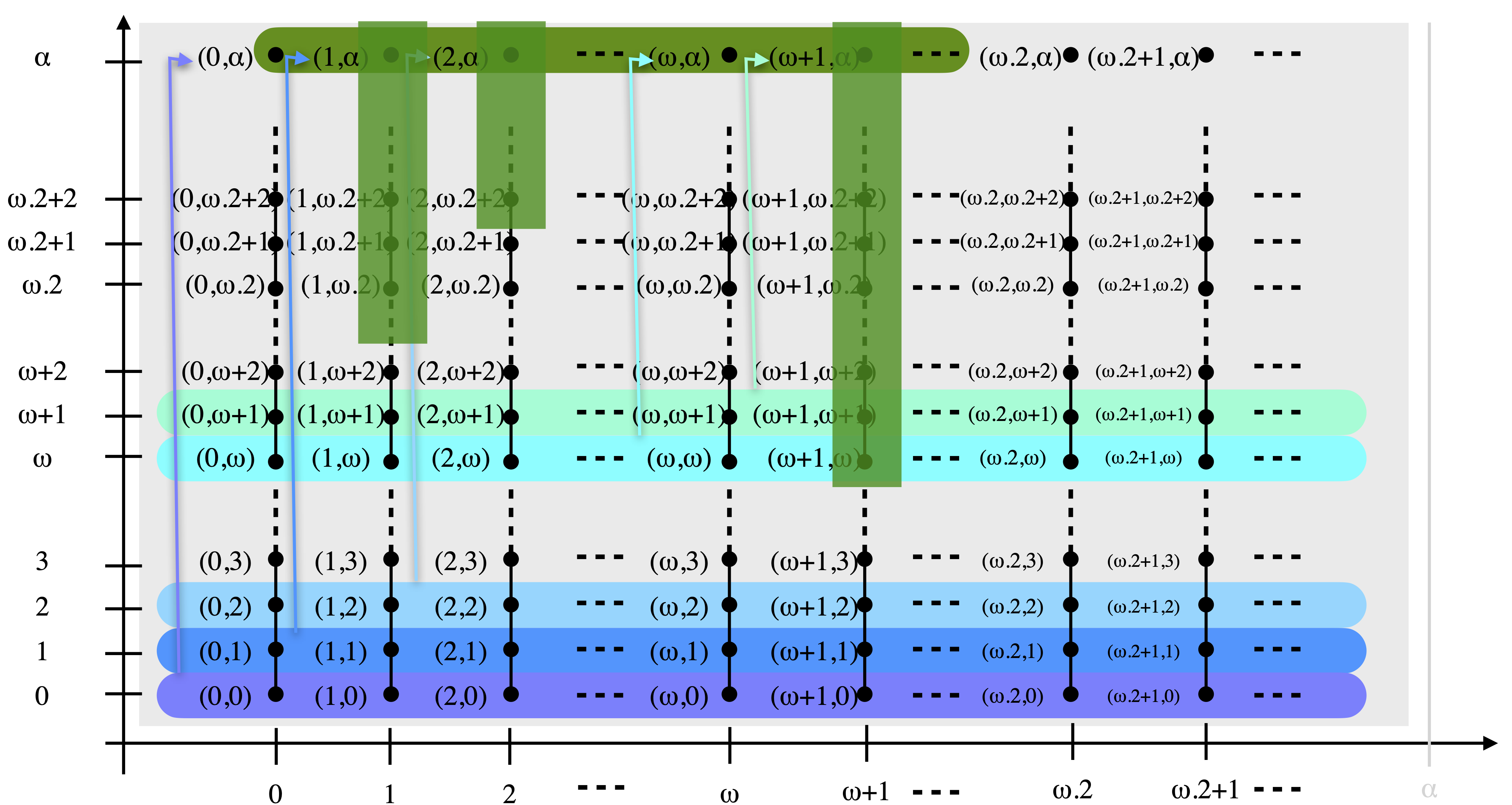
Hence, let Q be any compact saturated subset of Jα. The most important thing to look at is the set of points (j,α) of Q that lie on the top row Max Jα. In column j (i.e., below (j,α), vertically), it may be the case that there is just one point (necessarily (j,α)) in Q: in that case, we say that (j,α) is a singular point of Q. Otherwise, we say that (j,α) is a regular point of Q. The picture is as follows, where Q is depicted in green, there are three regular points (at the top of each of the three green columns), and the remaining green points on the top row are singular.
We inquire what the set of regular points of Q may be. The following argument only works if α>ω.
Proposition C. Let α be a regular ordinal. If α>ω, then Q can only have finitely many regular points.
Proof. The proof by Zhao, Xi, and Chen ([1], Lemma 2, item (2)) seems to be faulty, as noticed by Jordan Brown [3]. The result is correct, fortunately: Brown proposed a repaired proof [3, proof of Theorem 7.5, first paragraph], which we follow, only extending it from ω1 to the case of an arbitrary regular ordinal α>ω.
By contradiction, we assume that Q contains infinitely many regular points. Let (j0,α) be the first regular point of Q, namely the one where j0 is least. Since it is regular, there is a point (j0,k0) in Q, and we can again choose k0 to be minimal (hence <α, by definition of regular points). Next, we look for the second regular point (j1,α) of Q, namely such that j1 is minimal and strictly above j0; then there is a point (j1,k1), with k1 minimal (hence <α). Proceeding this way, we obtain an countably infinite sequence of points (jn,kn) in Q, where j0<j1<…<jn<…<α, and k0, k1, …, kn, …<α. See the following picture, where only three of those points are shown.
Let k=(supn∈N kn)+1. Since α>ω, and since α is a regular ordinal, we have supn∈N kn<α, and since α is a limit ordinal, it then follows that k<α. For every j<α, let Uj be the set consisting of the union of column number j with all the rows at and above row k, namely the set of points of the form (j, anything) or (anything, any ordinal ≥k). It is easy to see that Uj is Scott-open: see the following picture, where Uj is shown in orange.
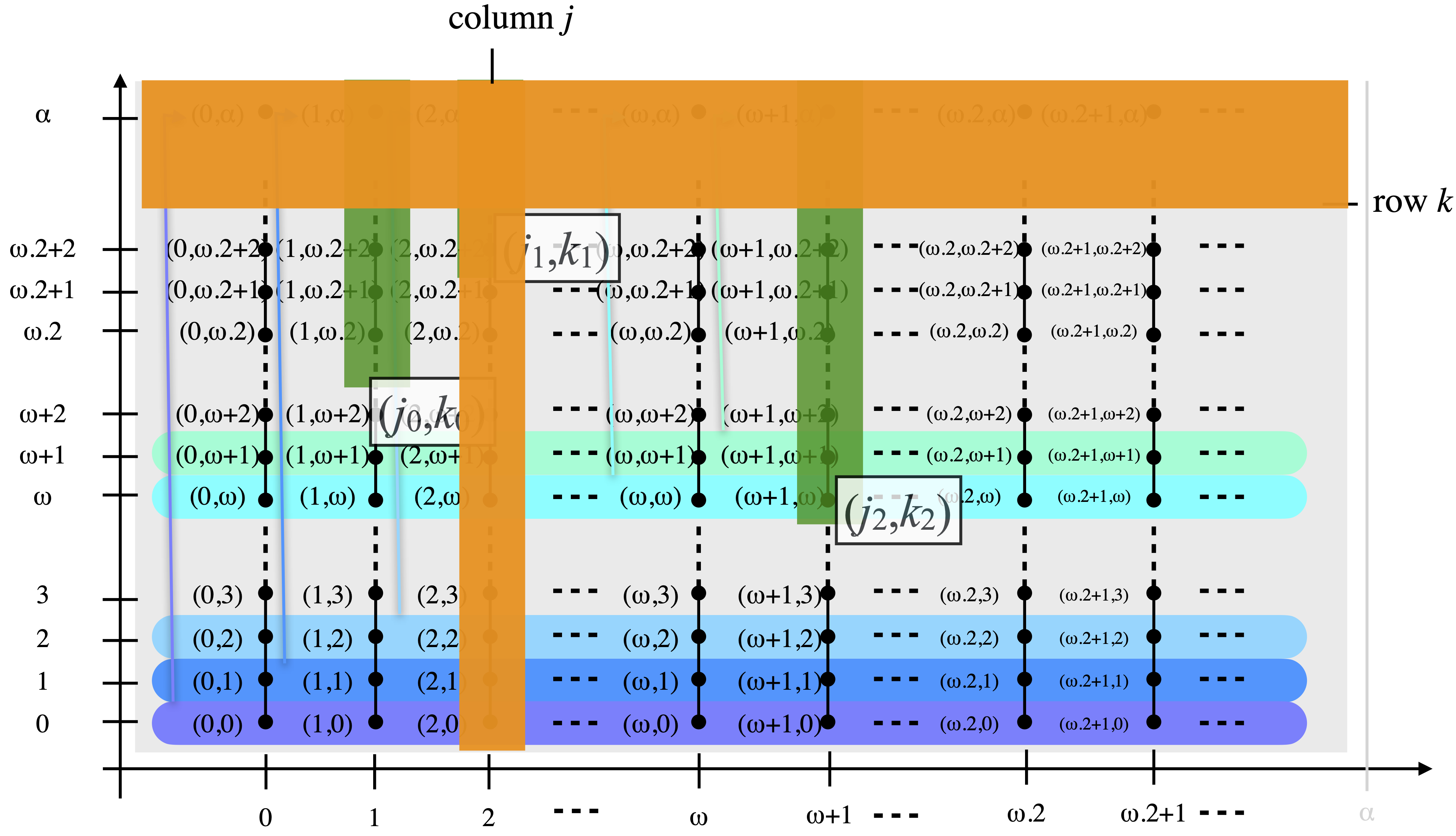
The sets Uj, j<α, form an open cover of Q, in fact of the whole of Jα. Since Q is compact, we can extract a finite subcover. But each Uj can contain at most one of the points (jn,kn): since kn<k, the only point (jn,kn) that can be in Uj must satisfy jn=j. It follows that the union of the sets Uj in the chosen finite subcover can contain only finitely many of the points (jn,kn), hence cannot contain Q: contradiction. ☐
Jα is well-filtered… when α>ω
Let α be a regular ordinal strictly larger than ω. We consider a filtered family (Qi)i ∈ I of compact saturated subsets of Jα, we let Q=∩i ∈ I Qi, and we assume that Q is included in some open subset U. In order to show that Jα is well-filtered, we need to show that some Qi is included in U.
We further define the preordering ⪯ on I by i⪯i’ if and only if Qi⊇Qi’. This will allow us to use phrases such as “for i large enough, …”, meaning “there is an index i0 ∈ I such that for every i ∈ I such that i0⪯i, …”.
The proof will now be done in three steps.
Step 1.
Proposition C tells us that every Qi contains only finitely many regular points. Since the set of regular points of Qi can only decrease or stay the same when i increases, and is finite, we obtain that it stays the same for i large enough.
Without loss of generality, we can restrict to the cofinal subfamily of those sets Qi. In other words, we assume that every Qi has exactly the same (finite) set of regular points. Let A be the finite set {j<α | (j,α) is a regular point of Qi}. That is now independent of i.
Step 2.
For each j in A, for every i in I, since (j,α) is a regular point of Qi, there is a point (j,k) such that k<α in Qi, and, as before, we can choose it lowest in column j: let kij be the ordinal k that we obtain this way.
For all i, i’ in I such that i⪯i’, we have kij≤ki’j. This is because ki’j is in Qi’, which is included in Qi; and then, (j,ki’j) is a point in column j of Qi, which is therefore above (j,kij). As a consequence, the family (kij)i ∈ I is a chain. Its supremum is in every Qi, hence in Q, hence in U, and since U is Scott-open, kij is in U for i large enough.
There are only finitely many indices j in A, so, for i large enough, all the points kij, j ∈ A, are in U. Hence, further restricting I to that cofinal family of indices i, we may assume that for every i in I, the points kij, j ∈ A, are all in U.
In other words, with that further restriction of I, the columns that pass through the regular points of Qi are included in U, for every i in I.
Step 3.
Let C be the complement of U. This is a closed set. Hence Qi ∩ C is compact, for every i in I. But, following Step 2, the columns that pass through the regular points of Qi are included in U, so Qi ∩ C only contains the singular points of Qi. In particular, Qi ∩ C is completely inside the top row Max Jα of Jα.
As such, Qi ∩ C is a compact subset of the space Max Jα. It is also saturated (upwards-closed) since Max Jα is T1 (it is a subset of maximal elements, hence all those elements are incomparable). Hence we have obtained a filtered family (Qi ∩ C)i ∈ I of compact saturated subsets of Max Jα. Moreover, its intersection is Q ∩ C, which is empty since Q is included in U.
Now recall from Proposition A’ that Max Jα is well-filtered. Hence, for some i in I, Qi ∩ C is empty. Equivalently, Qi is included in U. Done! Jα is well-filtered.
Since Jα is not sober (Fact 0), we have proved the following, which is [1, Theorem 1], [3, Theorem 7.5] in the special case where α=ω1.
Theorem. For every regular ordinal α>ω, Jα is a well-filtered, non-sober dcpo. ☐
As a final word, let me remind you that when α=ω, Jα=J is not sober, but is not well-filtered either (Exercise 8.3.9 in the book). Hence there is a curious gap between the case α=ω and the other cases!
- Dongsheng Zhao, Xiaoyong Xi, and Yixiang Chen. A new dcpo whose Scott topology is well-filtered but not sober. Topology and its Applications. Volume 252, 1 February 2019, Pages 97-102.
- Peter T. Johnstone. Scott is not always sober. Lecture Notes in Mathematics 871, Springer-Verlag, Berlin and New York, pages 282-283, 1981.
- Jordan Brown. Scott spaces and the dcpo category. The University of Chicago Mathematics REU 2019 (REU seems to mean Research Experience for Undergraduates).

— Jean Goubault-Larrecq (June 20th, 2020)
