The complete lattice Lfan (part II)

Last time, we had started to study the following poset L_fan, introduced independently in [3, 1, 4]: L_fan is N × N, with equality as ordering on the first component and the usual ordering on the second component, plus an extra bottom element ⊥, plus an extra top element ⊤:

We had seen that L_fan is a complete lattice with the Chen-Kou-Lyu property—in particular, (L_fan × L_fan)_σ = (L_fan)_σ × (L_fan)_σ, where the σ subscript means “with the Scott topology”—although it is neither core-compact nor first-countable (in its Scott topology).

The Scott topology on L_fan can be described explicitly. For every function f : N → N, let U_f be the subset of those pairs (m, n) of N × N such that n≥f(m), plus ⊤.

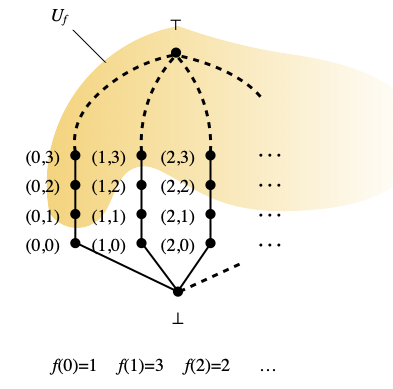
Then the Scott-open subsets of L_fan are exactly the sets U_f, where f ranges over the functions from N to N, plus the empty set, plus the whole space L_fan. Additionally, U_f ⊆ U_g if and only if f≥g, so that O(L_fan) is order-isomorphic to the poset (N → N)^op of functions from N to N, ordered with the opposite ordering ≥ (not ≤), with an additional bottom element (∅) and an additional top element (L_fan).

Sobriety

We start this month’s post with a nice piece of news about L_fan.

Lemma A. L_fan is sober in its Scott topology.

Proof. Let C be an irreducible subset of L_fan. It is non-empty, and downwards-closed, hence contains ⊥. If it only contains ⊥, then C is the downward closure of ⊥. If C contains ⊤, then C = ↓⊤. Henceforth, let us assume that C is neither of those. But C is closed, the closed sets are the complements of open sets, and we know what the open sets are: C is the complement of U_f, for some function f from N to N. Also, f is not the constant 0 function, since otherwise C would be {⊥}.

For every m ∈ N, let us say that C has a non-trivial column, and precisely that it has non-trivial column number m if and only if C contains a point (m, n) for some n, equivalently if and only if f(m)≠0. If so, there is a largest point (m, n) in C’s column m, namely (m, f(m)–1). Since f is not the constant 0 function, C has at least one non-trivial column. We claim that it has exactly one.

Otherwise, let us say that columns m and m’ are non-trivial, with m≠m’. Let g : N → N be as f, except that g maps m to f(m)–1, and let g’ be as f except that it maps m’ to f(m’)–1. Then C intersects U_g at (m, f(m)–1), U_g’ at (m’, f(m’)–1), but C does not intersect U_g ∩ U_g’, since the latter is equal to U_sup(g,g’) = U_f. This contradicts the fact that C is irreducible (see Trick 8.2.3 in the book).

Therefore C has exactly one non-trivial column, say with number m. Then C = ↓(m, f(m)–1). In all cases, C is the downward-closure of a (unique) point, so L_fan is sober. ☐

This was proved by Chen, Kou and Lyu [1, Example 4.13].

Weak Hausdorffness, and being a maximal limit space

The following properties were not considered by Chen, Kou and Lyu [1], or by Hertling [4], or by Xu, Shen, Xi and Zhao [3], but I think they deserve to be mentioned, specially considering that they were the topic of some of my recent posts.

A space X is weakly Hausdorff in the sense of Klaus Keimel and Jimmie Lawson [5] if and only if for any two points x and y in X, for every open neighborhood W of ↑x ∩ ↑y, there is an open neighborhood U of x and there is an open neighborhood V of y such that U ∩ V ⊆ W. I have already written a few times about that notion.

Lemma B. L_fan is weakly Hausdorff in its Scott topology.

Proof. We take two points x and y and an open neighborhood W of ↑x ∩ ↑y. The existence of U and V is obvious if x≤y (or symmetrically if y≤x): simply take the whole space for U and W for V. Hence let us assume that x and y are incomparable. Then none of x or y is equal to ⊤ or to ⊥, so we can write x as (m, n) and y as (m’, n’), where additionally m≠m’.

The intersection ↑x ∩ ↑y is then equal to {⊤}. If W is the whole space L_fan, then we can simply take U and V equal to the whole space as well. Henceforth, let us assume that W is a proper open subset of L_fan. Since W contains {⊤}, it is non-empty, hence of the form U_f, for some function f : N → N, by Lemma E. We let g : N → N be defined as f, except that it maps m to 0; and g’ be defined as f except that it maps m’ to 0. Then x = (m, n) is in U_g, y = (m’, n’) is in U_g’, and U_g ∩ U_g’ = U_sup(g,g’) = U_f = W, so we can take U ≝ U_g and V ≝ U_g’. ☐

In this post (March 2024) on Hoover’s maximal limit spaces [6], namely on spaces in which every convergent filter has a unique largest limit, we have shown (Theorem I there) that a maximal limit space is the same thing as a weakly Hausdorff, binary-bounded-sup-complete, monotone convergence space. By Lemma A, L_fan is sober, hence a monotone convergence space (see Proposition 8.2.34 in the book), it is weakly Hausdorff by Lemma B, and it is certainly binary-bounded-sup-complete, since it is a complete lattice, as we have seen last time. Therefore:

Proposition C. L_fan is a maximal limit space.

In particular, it is coherent, too. We will retrieve this below, when we study the compact saturated subsets of L_fan.

The dcpo O(L_fan) of Scott-open subsets of L_fan

The complete lattice O(L_fan) of Scott-open subsets of L_fan consist of the sets U_f, with f : N → N, ordered with the opposite of the pointwise ordering on functions f, plus a top element (the whole space L_fan), plus a bottom element (the empty set).

Let us write N_ω for the set of natural numbers N, with an extra element ω above all natural numbers. We order it in the usual way: 0 ≤ 1 ≤ … ≤ n ≤ … ≤ ω.

For every function g from N to N_ω, let dom g be the set of numbers m ∈ N such that g(m)≠ω. This is the domain of g, which I will write as dom g. We let FF be the set of functions f from N to N_ω whose domain is finite, ordered pointwise. Hertling [4, before Lemma 4.13] uses actual total functions from a finite subset of N to N (not N_ω), but I think this definition of FF will be more practical.

For every g ∈ FF, let V_g be the collection of Scott-open subsets of L_fan of the form U_f with f≤g, plus L_fan itself. The following is due to Hertling [4, Lemma 4.14].

Proposition D. The sets V_g, g ∈ FF, plus {L_fan} and O(L_fan), form a base of the Scott topology on O(L_fan).

Proof. We first check that V_g is Scott-open. It is clear that V_g is upwards-closed; notably, if U_f is in V_g, then f≤g, so every U_f’ containing U_f must be such that f’≤f (that was Fact F of last time), whence U_f’ is in V_g, too. We consider any directed family D of Scott-open subsets of L_fan whose supremum is in V_g, and we wish to show that D intersects V_g. If L_fan ∈ D, then some element of D (L_fan itself) is in V_g, and we are done. Hence we assume that L_fan is not in D. It cannot be the case that D only contains the empty set, since sup D would be the empty set, and ∅ is not in V_g. Then we may assume that D does not contain the empty set, without loss of generality: removing the empty set yields a cofinal subfamily, which will in particular be directed and have the same supremum. This way, we may assume that D is a directed family of sets of the form U_fi, i ∈ I. Using the order-isomorphism with (N → N)^op plus extra top and bottom elements (Fact F of last time), its supremum is U_f where f is the pointwise infimum of (f_i)_{i ∈ I}.

By assumption, U_f is in V_g, so f≤g. For each one of the elements m of the domain of g, we can then find an index i ∈ I such that f_i(m)≤g(m); indeed, the family (f_i(m))_{i ∈ I} is well-founded, namely it reaches its least element. Since dom g is finite and (f_i)_{i ∈ I} is directed (with respect to ≥), we can take the same i ∈ I for every m ∈ dom g; namely, for every m ∈ dom g, f_i(m)≤g(m). For every natural number m outside dom g, we have g(m)=ω, so f_i(m)≤g(m) as well. Therefore f_i≤g. This terminates our argument that V_g is Scott-open.

Second, O(L_fan) is trivially Scott-open, and {L_fan} is Scott-open, too: given any directed family of Scott-open subsets (U_i)_{i ∈ I} of L_fan whose union is in {L_fan}, namely whose union is equal to L_fan, or equivalently, whose union contains ⊥, some U_i must contain ⊥ already, namely U_i=L_fan.

Finally, we need to show that the set V_g, {L_fan} and O(L_fan) form a base of of the Scott topology on O(L_fan). Let U ∈ O(L_fan), and let W be a Scott-open subset of O(L_fan) such that U ∈ W. If U=L_fan, then U ∈ {L_fan} ⊆ W. If U=∅, then W=O(L_fan). It remains to see what happens when U = U_f for some function f from N to N. For every k ∈ N, let g_k : N → N_ω map every natural number m<k to f(m), and all others to ω. Clearly, U = U_f ∈ V_gk for every k ∈ N (since f≤g_k), and we claim that V_gk ⊆ W for some k ∈ N; this will finish the proof.

Let us assume that V_gk is not included in W for any k ∈ N. For every k ∈ N, there is an element of V_gk that is not in W. That element cannot be L_fan, since L_fan is in W (W is non-empty since it contains U, and upwards-closed, hence contains the largest element of O(L_fan)). It cannot be the empty set either, since the empty set is not in V_gk. Hence it must be equal to U_fk for some function f_k : N → N. For the record, we will to remember that: (a) U_fk ∈ V_gk and: (b) U_fk ∉ W.

Let f’ : N → N map every number m to max(f(m), f₀(m), …, f_m(m)). Then f≤f’, and: (c) f_k(m)≤f’(m) for every m≥k.

For every k ∈ N, we now define f’_k : N → N as mapping every number m<k to f(m) and every number m≥k to f’(m). Then f’ = f’₀ ≥ f’₁ ≥ … ≥ f’_k ≥ …, and inf_{k ∈ N} f’_k = f. Using the order-isomorphism with (N → N)^op plus extra top and bottom elements (Fact F of last time), U_f’ = U_f’0 ⊆ U_f’1 ⊆ … ⊆ U_f’k ⊆ …, and sup_{k ∈ N} U_f’k = U_f. Since the latter is in W, which is Scott-open, some U_f’k is in W.

We claim that f’_k ≥ f_k. For every m≥k, f’_k(m) = f’(m) ≥ f_k(m), by (c) (see a few lines back). For every m<k, f’_k(m) = f(m); since U_fk is in V_gk (by (a)), f_k≤g_k, so f_k(m)≤g_k(m), but since m<k and by definition of g_k, g_k(m)=f(m); so f’_k(m) = f(m) = g_k(m) ≥ f_k(m).

We can now conclude: f’_k ≥ f_k, so by the usual isomorphism (Fact F of last time), U_f’k is included in U_fk; since U_f’k is in W and W is upwards-closed, U_fk is in W, too. But that contradicts the definition of U_fk, namely (b). Therefore V_gk ⊆ W for some k ∈ N, as promised. ☐

As a side remark, the base given in Proposition D is countable. Therefore, and while L_fan is not first-countable hence not second-countable, O(L_fan) is second-countable in its own Scott topology, as was noted by Hertling.

L_fan is consonant

A space X is consonant if and only if for every open subset U of X, for every Scott-open neighborhood W of U in OX, there is a compact saturated subset Q of X such that Q ⊆ U and every open neighborhood of Q in X belongs to W. I have talked quite a few times about that property. Let me recall that every locally compact space is consonant (Exercise 5.4.12 in the book), and that every regular Čech-complete space is consonant (the Dolecki-Greco-Lechicki theorem, see Exercise 8.3.4 in the book).

Lemma E. Every sober space X such that OX has a base of Scott-open subsets U that are filters (viz., [upwards-closed, non-empty and] such that the intersection of any two elements of U is in U) is consonant.

Proof. Let U be an open subset of X, and W be a Scott-open neighborhood of U in OX. By assumption, there is a Scott-open neighborhood U of U included in W that is a filter. By the Hofmann-Mislove theorem (Theorem 8.3.2 in the book), since X is sober, U is the collection of open neighborhoods of some compact saturated subset Q of X. This is the desired set Q. ☐

The sets V_g, with g ∈ FF, are filters. Given any two open subsets in V_g, if one is equal to the whole of L_fan, then their intersection is the other one, which is in V_g; otherwise, they are of the form U_f and U_f‘ for some functions f, f’ : N → N such that f, f’ ≤ g, and by the order-isomorphism with (N → N)^op plus a top and a bottom element (Fact F of last time), their intersection is U_max(f,f’); since max(f,f’)≤g, U_max(f,f’) is also in V_g. It is easy to see that {L_fan} and O(L_fan) are filters, too. Those form a base of the Scott topology on O(L_fan), by Proposition D. Since L_fan is sober (Lemma A), we can apply Lemma E, and we obtain the following.

Proposition F. L_fan is consonant in its Scott topology.

The compact saturated subsets of L_fan

Given any function g ∈ FF, let Q_g be the subset of L_fan consisting of ⊤ plus all pairs (m, n) where m ∈ dom g and n≥g(m). Hence Q_g is defined just like the Scott-open set U_f, except that g is a slightly different kind of function; also, while both Q_g and V_g are indexed by the same kind of functions g, V_g was a Scott-open subset of O(L_fan), while Q_g will be a compact saturated subset of L_fan. There is a link, though: V_g is the collection of open neighborhoods of Q_g in L_fan.

Here is an illustration of what Q_g looks like, with a function g of domain {0, 2}:

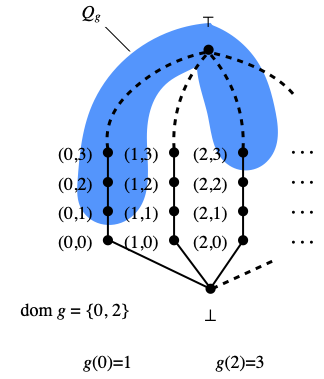
Lemma G. The compact saturated subsets of L_fan are the empty set, the whole space L_fan and the sets of the form Q_g, where g ranges over FF. They are all finitary compact, namely, upward closures of finite sets.

Proof. All those sets—the empty set, L_fan, Q_g—are finitary compact; notably, Q_g is the upward closure of the finite set of points (m, g(m)), m ∈ dom g if dom g is non-empty, and is the upward closure of ⊤ if dom g is empty. Therefore they are all upwards-closed and compact.

Conversely, let Q be a non-empty proper compact saturated subset of L_fan. Being proper, it does not contain ⊥. Since it is non-empty, it contains ⊤. For every m ∈ N, let us say that column m is empty if and only if Q contains no pair (m, n) for any n (but for this particular value of m); it is non-empty otherwise.

Let I be the set of indices m ∈ N such that column m is non-empty. There is a smallest natural number n such that (m, n) is in Q, and we call it g(m). For every number m outside I, we let g(m) ≝ ω. This defines a function g from N to N_ω, whose domain is I.

By way of contradiction, let us assume that I is infinite. We build a function f_m : N → N, one for each m ∈ I, which maps m to g(m), every m’ ∈ I–{m} to g(m’)+1, and all other indices to some arbitrary value, say 0. The Scott-open sets U_fm, m ∈ I, form an open cover of Q: every point of Q (other than ⊤, which is in any U_fm anyway—and there is such a U_fm, since I is non-empty) is of the form (m, n) with n≥g(m)=f_m(m), hence is in U_fm. That open cover has no strict subcover: if we remove any set U_fm (m ∈ I) from the cover, the point (m, f(m)) of Q fails to be in any U_fm’, m’ ∈ I–{m}. Since I is infinite, this contradicts the fact that Q is compact.

Hence I is finite. Therefore g is in FF, and it is easy to see that Q=Q_g. ☐

Fact H. For all g, g’ ∈ FF, Q_g ⊇ Q_g’ if and only if g ≤ g’.

With this characterization, we retrieve some results we have already obtained, in a more concrete fashion:

• L_fan is not core-compact. Indeed, L_fan is sober (Lemma A) and in that case, core-compactness is equivalent to local compactness (see Theorem 8.3.10 in the book). But the only compact saturated subset of L_fan with non-empty interior is L_fan itself. Indeed, the proper compact saturated subsets are of the form Q_g, and none contains any Scott-open subset of the form U_f. Explicitly, U_f ⊆ Q_g if and only if f ≥ g, and that cannot happen for an N-valued function f and a function g in FF.
• L_fan is coherent. This is a consequence of the fact that L_fan is a maximal limit space, which we have seen in Proposition C. More directly, the intersection of Q_g and of Q_g’ is equal to Q_h, where h maps every m ∈ N to max(g(m), g’(m)).

The Smyth hyperspace of L_fan

Last time (Corollary C), we had mentioned that, because L_fan has the Chen-Kou-Lyu property, the Scott topology coincides with the lower Vietoris topology on the Hoare hyperspace H(L_fan). Having explored the shape of compact saturated subsets of L_fan, we can now investigate the other classical hyperspace, the Smyth hyperspace.

The Smyth hyperspace QX of a space X is the space of all compact saturated subsets of X, with the upper Vietoris topology: its basic open subsets are of the form ☐U ≝ {Q ∈ QX | Q ⊆ U}, where U ranges over the open subsets of X.  QX is a T₀ space, whose specialization ordering is reverse inclusion. With what we know about the compact saturated subsets of L_fan, we can show the following.

Proposition I. The upper Vietoris topology coincides with the Scott topology of ⊇ on Q(L_fan) (where L_fan is given its Scott topology).

Proof. First, every upper Vietoris open subsets of Q(L_fan) is Scott-open. Indeed, since L_fan is sober (Lemma A), hence well-filtered (Proposition 8.3.5 in the book), the supremum of any directed family (Q_i)_i∈I in Q(L_fan) is its intersection ∩_i∈I Q_i (Proposition 8.3.25 in the book), and ∩_i∈I Q_i is in a basic open set ☐U if and only if some Q_i is in ☐U already (this is well-filteredness).

Second, let U be any Scott-open subset of Q(L_fan). For every Q ∈ U, we will find a Scott-open subset V of L_fan such that Q ∈ ☐V ⊆ U. By Lemma G, Q may have one of three possible forms. If Q=L_fan, then U is the whole of Q(L_fan), since U is upwards-closed and Q is the least element of Q(L_fan); then we may define V as L_fan. If Q is empty, then we may take V ≝ ∅. In the case that remains, Q = Q_g for some unique g ∈ FF, and we build a sequence of functions g_n in FF by induction on n ∈ N, as follows. The invariants we will maintain are:

• (A) Q_gn is in U;
• (B) the domain of g_n+1 is equal to the domain of g_n plus the one-element set {k_n}, where (k_n)_n∈N is a fixed enumeration without repetition of those natural numbers that lie outside the domain of g.

We start by defining g₀ as g. Given that we have defined g_n, we observe that the functions g_n[k_n ↦ m], defined just as g_n except that k_n (which is outside the domain of g_n) is mapped to the natural number m, form a monotone sequence when m varies over N, and that their supremum is g_n. Through the order-isomorphism stated in Fact H, Q_gn is the supremum of the monotone sequence of compact saturated sets Q_{gn[kn ↦ m]}, m ∈ N. Since Q_gn is in U and U is Scott-open, Q_{gn[kn ↦ m]} is in U for some m ∈ N. We pick one such m, for example the least one, and we define g_n+1 as g_n[k_n ↦ m]. Hence Q_gn+1 is in U (see (A)) and (B) is true.

Now that we have defined the functions g_n, we define f : N → N as the pointwise infimum of g_n, n ∈ N. There is a simpler way of describing f: for every m ∈ N, g_n(m) may be equal to ω for small values of n, but for any n large enough, g_n(m) will be the same natural number, independently of n; and that number is f(m). This is a consequence of (B).

We let V ≝ U_f. This is Scott-open in L_fan. Also, Q = Q_g is included in U_f, hence is an element of ☐V. Indeed, ⊤ is in U_f, and all the other elements of Q_g are pairs (m, n) of natural numbers with n≥g(m); since n is a natural number, g(m)≠ω, so m is in the domain of g=g₀, and therefore g(m)=f(m), whence n≥f(m).

Finally, we claim that ☐V ⊆ U. Let Q’ be any element of ☐V. Q’ cannot be the whole of L_fan, since otherwise ⊥ would be in Q’, hence in V = U_f, and that is impossible. If Q’ is empty, then certainly it is in U, because U is upwards-closed, non-empty (U contains Q) and therefore contains the top element of Q(L_fan), which is the empty set. Finally, and using Lemma G, the only case that remains is that Q’ would be of the form Q_g’ for some function g’ in FF. Since Q’ = Q_g’ ⊆ V = U_f, every pair (m, n) such that n≥g’(m) satisfies n≥f(m), so g’≥f. Since the domain dom g’ of g’ is finite, and since the domains of the functions g_n form a monotone sequence of sets dom g ∪ {k₀, …, k_n–1} as n varies, and whose union is the whole of N, it must be the case that dom g’ ⊆ dom g_n for some n large enough. But f coincides with g_n on dom g_n, so g’≥f entails that g’≥g_n: for every m in dom g’ ⊆ dom g_n, g’(m)≥f(m)=g_n(m), and for every m outside dom g’, g’(m)=ω. By Fact H, Q_g’ lies above (namely, is included in) Q_gn. But, by (A), Q_gn is in U. Since U is upwards-closed, Q_g’ (= Q’) is in U. Since Q’ is arbitrary in ☐V, this shows that ☐V ⊆ U. ☐

Xiaoyong Xi calls conformal any well-filtered topological space X such that the upper Vietoris and Scott topologies of ⊇ coincide on QX. (I am not sure where this comes from. He gave me some names for the inventors of the notion, but I was unable to find a corresponding reference.)

One can read Lemma 8.3.26 in the book as saying that every well-filtered, locally compact space is conformal. A theorem due to Xiaoquan Xu and Zhongqiang Yang [9, Corollary 5.9] states that every well-filtered, first-countable space such that every compact saturated subset has countably many minimal elements is conformal. Here L_fan is neither core-compact nor first-countable, but is conformal nonetheless. (Note that its compact saturated subsets not only have countably many, but even finitely many minimal elements.)

I will conclude by the following summary: L_fan is an example of a conformal space in its Scott topology, which is not first-countable and not core-compact, but is a consonant, maximal limit space and a complete lattice.

1. Yu Chen, Hui Kou, and Zhenchao Lyu.  Two topologies on the lattice of Scott closed subsets.  Topology and its Applications, 306, 107918, 2022.
2. Hualin Miao, Xiaoyong Xi, Qingguo Li, and Dongsheng Zhao. Not every countable complete distributive lattice is sober, Mathematical Structures in Computer Science 33(9):809–831, October 2023. arXiv version arXiv:2205.00250v1 [math.GN], 30 Apr 2022.
3. Xiaoquan Xu, Chong Shen, Xiaoyong Xi, and Dongsheng Zhao. First countability, ω-well-filtered spaces and reflections.  Topology and its Applications, 279, 107255, 2020.
4. Peter Hertling.  Topological properties of the binary supremum function. Semigroup Forum 104:618–646, 2022.
5. Klaus Keimel and Jimmie Lawson. Measure extension theorems for T₀-spaces. Topology and its Applications, 149(1–3), 57–83, 2005.
6. Douglas N. Hoover.  Maximal Limit Spaces, Powerspaces, and Scott Domains.  Proceedings of the 11th International Conference on Mathematical Foundations of Programming Semantics (MFPS), New Orleans, Louisiana, USA. Electronic Notes in Theoretical Computer Science 1:253–272, 1995.
7. Reinhold Heckmann. An upper power domain construction in terms of strongly compact sets. In: Brookes, S., Main, M., Melton, A., Mislove, M., Schmidt, D. (eds) Mathematical Foundations of Programming Semantics. MFPS 1991. Lecture Notes in Computer Science, vol 598. Springer, Berlin, Heidelberg. https://doi.org/10.1007/3-540-55511-0_14
8. Andrea Schalk. Algebras for Generalized Power Constructions. PhD Thesis, TU Darmstadt, 1993.
9. Xiaoquan Xu and Zhongqiang Yang.  Coincidence of the upper Vietoris topology and the Scott topology.  Topology and its Applications 288, February 2021.

— Jean Goubault-Larrecq (June 20th, 2024)